This week's Puzzle - What can you see in this picture?
The puzzle of 2 weeks ago had many of you scratching your heads. Only Susanna and Hashim were spot on to solve this problem. Grandson Samuel was close but did not know how to express the answer as he has not done geometry so as to use words as hypotonuse, areas, and quadilaterals.
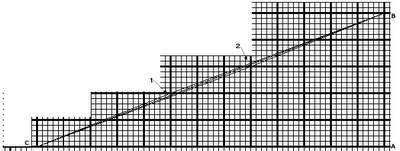
Look very carefully at the picture above.
If a straight line is drawn between B and C, the area of the triangle is 32.5 sq. x.
If the large Red Triangle is at the bottom left, then a straight line cannot be drawn between B and C which will pass through the vertex of that triangle. At the Point 2 on the graph, there will be a kink, and the area of the whole quadilateral is 32 sq. x.
If the large Red Triangle is at the top right, then again it is not possible to draw a straight line between B and C which passes through the vertex of the smaller green triangle. It will change at Point 1 and the area of the whole quadilateral is 33 sq. x.
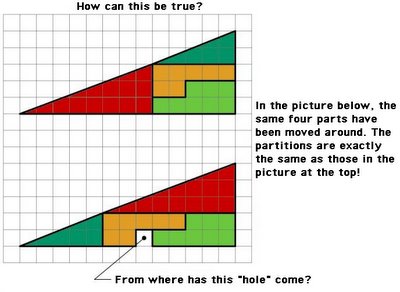
So the difference between the top and bottom figures is exactly 1 sq. x, which is the extra square that you see in the bottom figure.
This is masked in the picture by the "thickness" of the line drawn between B and C.
Very few of you actually sat down and worked the areas of each of the blocks and the whole triangle. This would have shown a difference of exactly 1 sq. x. between the top and bottom figures, with the straight line between B and C giving the area as 32.5 sq. x.
And here is an easy one. Count how many "black" dots are in this picture.

No comments:
Post a Comment